
The StatsCircularMoments operation can be used as the WaveStats operation for circular data. You can also use the operation to perform uniformity tests or to convert periodic data of various formats to waves containing angles in radians.
Data Conversion Examples
Consider the date data in the wave dateData1 can be converted into an angle wave (in radians) using the StatsCircularMoments operation with /MODE=5. To run the operation execute the command:
StatsCircularMoments/T=1/Q /SAW/Mode=5/KUPR/RAYL dateData1
| dateData1 | W_AngleWave | |
| 12/25/04 20:58:45 | 5.06708 | |
| 1/21/05 08:31:08 | 4.25635 | |
| 1/2/05 07:53:44 | 0.405367 | |
| 12/18/04 14:01:59 | 3.6483 | |
| 1/28/05 01:50:50 | 5.67514 | |
| 1/14/05 19:02:46 | 2.83757 | |
| 1/18/05 21:32:49 | 3.6483 | |
| 1/19/05 13:36:46 | 3.85098 | |
| 1/8/05 00:05:24 | 1.62147 | |
| 12/9/04 13:05:50 | 1.82415 | |
| 12/26/04 15:18:29 | 5.26977 | |
| 12/17/04 07:26:29 | 3.44562 | |
| 12/4/04 00:37:08 | 0.810734 | |
| 12/28/04 02:32:47 | 5.67514 | |
| 1/13/05 08:02:51 | 2.63488 | |
| 12/18/04 19:17:53 | 3.6483 |
Besides converting the date into circular data, the operation also performed the Kuiper and Rayleigh tests for uniformity. The results are stored in the wave W_CircularStats. The first part of the table represents various measures of circular statistics:
| number_of_points | 16 |
| number_of_NaNs | 0 |
| C | -2.7804 |
| S | -2.36277 |
| R | 3.64874 |
| cBar | -0.173775 |
| sBar | -0.147673 |
| rBar | 0.228046 |
| tBar | 3.84597 |
| V | 0.771954 |
| v | 1.71942 |
| median | 3.6483 |
| mean_deviation | 1.22877 |
| Circular_Dispersion | 8.75803 |
| Skewness | -0.0902807 |
| Kurtosis | 0.10402 |
The second part of the table contains the results of the Kuiper and Rayleigh tests:
| Kuiper_V | 1.13873 |
| Kuiper_Critical | 1.74726 |
| Kuiper_P_Value | 0.627286 |
| Rayleigh_Z | 0.832081 |
| Rayleigh_CriticalZ | 2.94819 |
| Rayleigh_significance_prob | 0.441939 |
Both tests indicate that H0 (uniform distribution) hypothesis can't be rejected.
Axial data
Here the input is cyclical data as shown below:
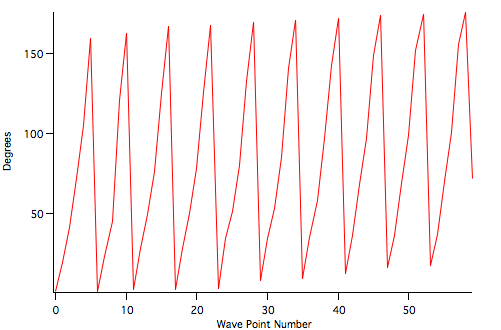
To run the tests execute the following command:
StatsCircularMoments/mode=2/KUPR/RAYL/T=1/Q/AXD=2 axddata
The operation multiplies the data in axdData a factor of 2 (/AXD=2) and handles the input as if it contained angles in degrees (/mode=2).
The results in the "Circular Stats" table are:
| number_of_points | 60 |
| number_of_NaNs | 0 |
| C | 10.9331 |
| S | 9.09466 |
| R | 14.2213 |
| cBar | 0.182218 |
| sBar | 0.151578 |
| rBar | 0.237022 |
| tBar | 0.693861 |
| V | 0.762978 |
| v | 1.69682 |
| median | 0.74489 |
| mean_deviation | 1.277 |
| Circular_Dispersion | 9.08257 |
| Skewness | 0.030089 |
| Kurtosis | 0.00192745 |
| Confidence_d | 0.848389 |
| Kuiper_V | 1.58639 |
| Kuiper_Critical | 1.74726 |
| Kuiper_P_Value | 0.118182 |
| Rayleigh_Z | 3.37076 |
| Rayleigh_CriticalZ | 2.98324 |
| Rayleigh_significance_prob | 0.0336911 |
These results are rather interesting in that the Kuiper test does not reject uniformity while the Rayleigh test does.

Forum

Support
Gallery
Igor Pro 9
Learn More
Igor XOP Toolkit
Learn More
Igor NIDAQ Tools MX
Learn More